Geometry - Quantitative Aptitude
Q1. If an angle is its own complementary angle, then its measure is
(a) 300
(b) 450
(c) 750
(d) 900
Answer: (b) 450
Let, the measure of the angle be x0
Now x0 = 900 - x0
⇒ 2x0 = 900
⇒ x0 = 450
Q2. The supplement of 800 is
(a) 600
(b) 750
(c) 900
(d) 1000
Answer: (d) 1000
Supplement of 800 = 1080 - 800 = 1000
Q3. Δ ABC is right angled at B. If AB = 10 cm, BC = 5 cm then AC = ?
(a) 3√2 cm
(b) 3√5 cm
(c) 5√5 cm
(d) 5√7 cm
Answer: (c) 5√5 cm
We have AC2 = AB2 + BC2 = 102 + 52
AC2 = 100 + 25
⇒ AC2 = 125
⇒ AC = √125 = 5√5
Q4. The angles of a traingle are 4x0, (2x - 9)0 and (7x - 6)0. The value of x is
(a) 150
(b) 300
(c) 450
(d) 600
Answer: (a) 150
We have
4x0 + (2x - 9)0 + (7x - 6)0 = 1800
⇒ 4x0 + 2x0 - 90 + 7x0 - 60 = 1800
⇒ 13x0 = 1800 + 90 + 60 = 1950
⇒ x0 = 1950/13 = 150
∴ x = 150
Q5. The perimeters of two simillar triangles Δ ABC and Δ PQR are 36 cm and 24 cm respectively. If PQ = 10 cm then AB = ?
(a) 15 cm
(b) 30 cm
(c) 45 cm
(d) 90 cm
Answer: (a) 15 cm
Δ ABC ∼ Δ PQR
| ∴ | AB | = | BC | = | CA | = | AB + BC + CA | = | 36 |
| PQ | QR | RP | PQ + QR + RP | 24 |
| ∴ | AB | = | 36 |
| PQ | 24 |
| ⇒ | AB | = | 3 |
| 10 | 2 |
⇒ AB = (3/2) X 10 cm = 15 cm
Q6. In the given figure, what is the value of y, if 3x = 5y
(a) 52.50
(b) 59.50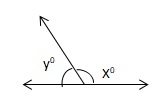
(c) 67.50
(d) 72.50
Answer: (c) 67.50
We have
x + y = 1800
⇒ 3x + 3y = 3 X 1800
⇒ 5y + 3y = 5400
⇒ 8y = 5400
⇒ y = 67.50
Q7. The sum of all angles around a point is
(a) 00
(b) 1800
(c) 2700
(d) 3600
Answer: (d) 3600
Q8. In Δ ABC, AB = AC, ∠A = x0, ∠B = (x - 15)0, then what is the value of x
(a) 450
(b) 600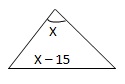
(c) 700
(d) 1200
Answer: (c) 700
We have
∠ B = ∠ C
⇒ (x - 15)0 = ∠ C
A/Q ∠ A + ∠ B + ∠ c = 1800
⇒ x + x - 150 + x - 150 = 1800
⇒ 3x = 1800 + 300 = 2100
⇒ x = 700
Q9. A chord AB of length 20 cm is at a distance of 4 cm from the center O of a circle. The radius of the circle is
(a) 2√21 cm
(b) 2√29 cm
(c) 3√12 cm
(d) 3√31 cm
Answer: (b) 2√29 cm
We have
AB = 20 cm
OL = 4 cm
AL = 20/2 cm = 10cm
Now OA2 = AL2 + OL2 = 102 + 42 = 116 cm2
∴ OA = √116 cm2 = 2√29 cm
Q10. In a Δ ABC, if 2∠A = 3∠B = 6∠c, then ∠A = ?
(a) 450
(b) 900
(c) 1100
(d) 1450
Answer: (b) 900
We have
∠A + ∠B + ∠C = 1800
⇒ ∠A + 2/3∠A + 2/6∠A = 1800
| ⇒ | 6∠A + 4∠A + 2∠A | = 1800 |
| 6 |
⇒ 12∠A = 1800 X 6
⇒ ∠A = 900

Practice Test Exam